Perpendicularity: key to machining good parts

For a good part of my career, I thought of squareness—or, more correctly, perpendicularity—as a visual geometry. Many of the tools I use to inspect right angles are comparative and involve eyeballing, so it is natural to think of perpendicularity in visual terms.

Checking perpendicularity is a recurring theme on the shop floor—one that deserves detailed discussion and special tools.

The right angle is an invention of human mechanical arts. Its presence in nature is accidental and not by design. Perpendicularity is also foundational to the mechanical arts, so it is crucial to be able to measure perpendicularity with certainty.

Figure 1. Tool and die makers have at their disposal simple, elegant tools for squaring parts to tight tolerances. Images courtesy of T. Lipton.

The closer the limits are that you need to work with, the more important it is to quantify the magnitude of the observed error. High-quality measurement feedback is necessary to produce first-class work. If you cannot verify your output, you never will be able to produce superior work.

So, you might wonder, “How square is that really nice machinist square I use to check my work?” The answer became obvious to me when I started to make parts and gages near the limits of my measuring abilities and found that some of my established references were not to be trusted.

The geometric dimensioning and tolerancing definition of perpendicularity is something like this: The surface must be perpendicular within X tolerance, with respect to the datum determined in the feature control frame. So, in reality, you need to know how far out of square a particular surface is to a specific dimension. Eyeballing is not good enough anymore.

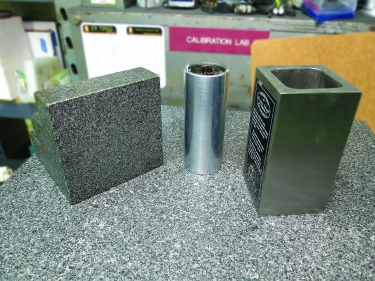
Figure 2. Normally, commercial and modified surface gages, which are sometimes called squareness comparators, are set using a master square reference, such as a cylindrical square, or a known calibrated right angle.